Superfluid Onset and Prewetting of 4He on Rubidium

J. A. Phillips, D. Ross, P. Taborek and J. E. Rutledge 
Department of Physics and Astronomy 
University of California, Irvine 92697 

(1998)
 
 
Abstract 

Introduction 

Experimental Methods 

Data 

Discussion 

Conlcusions 

References 
 
 
 
 

Projects Page 
 
 
 
 

Data

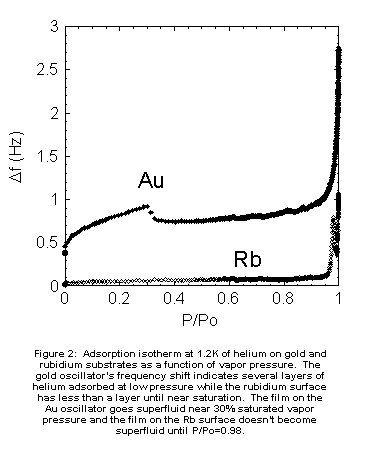figure 2In order to cover a large region of the m-T plane, isotherms were performed from T=0.15K to 2.2K in the pressure range from vacuum to liquid-vapor coexistence. Figure 2 shows a frequency shift isotherm at 1.2K for both the Au and the Rb-coated oscillators. Note that the Au oscillator has a frequency shift corresponding to several adsorbed layers even at very low pressures, while the Rb oscillator shows less than one half monolayer adsorption until quite near the saturated vapor pressure. In subsequent figures, we will usually omit the low coverage, low pressure regime and concentrate on the region near coexistence where the film thickness changes rapidly.  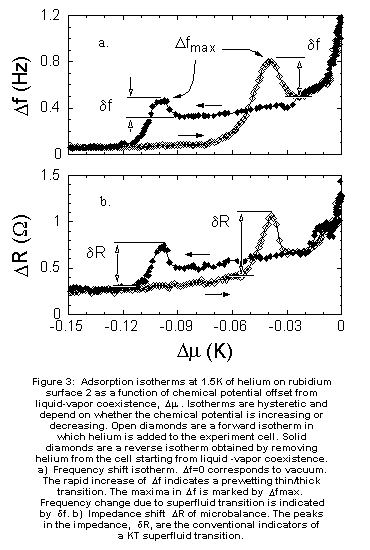In Figure 3 we present a sample pair of isotherms performed at 1.50K on surface 2. The figure shows the resonant frequency shift, Df, and dissipation change, DR, as a function of Dm. The system started in vacuum and gas was slowly added to the experiment cell. This type of isotherm in which  Dm increases as a function of time is referred to as a “forward” isotherm and is indicated by the open diamonds in Figure 3.   At low chemical potential Fig. 3a indicates that at most only 0.2 monolayers are adsorbed. Near Dm = -0.05 K, Df abruptly increases. This behavior is characteristic of a prewetting thick/thin transition. When the frequency shift reaches a value of 0.8 Hz (corresponding to a thickness of 4 layers), the film undergoes a superfluid transition. This transition is identified by two significant features: Df drops as the superfluid fraction uncouples from the oscillator and DR has the predicted dynamic KT peak at the same Dm (Figure 3b). As more helium is added to the cell, Df continues to increase because the film thickness is rapidly increasing and the oscillator has some non-transverse motion which couples to the superfluid film. Since the film ends up in a thick state at saturation, it is said to wet the rubidium. 
figure 3We have systematically looked for hysteresis in the thin normal -> thick superfluid transition by measuring “reverse” isotherms in which small amounts of helium gas are sequentially removed from the experiment cell. In Figure 3, the reverse isotherm, denoted by solid diamonds, starts at coexistence in the thick superfluid state and is coincident with the forward isotherm down to Dm = -0.03 K, where the two sets of data diverge. On the reverse isotherm, the film remains superfluid until Dm = -0.10 K, where the film first becomes normal (Df and DR increase) and then goes through the prewetting transition to the thin state. 
The data shown in Fig 3 are typical of our data between 0.75 K and 1.95 K. Throughout this range the forward and reverse Dm values at prewetting and superfluid onset are strongly hysteretic. In addition, the superfluid onset thicknesses, the onset superfluid jumps, and the dissipation peak heights and areas are larger on the forward branch at each temperature. At 1.95 K the hysteresis abruptly decreases and the forward and reverse isotherms become nearly identical. Below 1.95 K the difference between the prewetting and superfluid onset Dm values is small and roughly temperature independent. Above 1.95 K the Dm difference rapidly grows, and prewetting and superfluid onset become clearly distinguishable transitions. 
Isotherms from three rubidium substrates in the temperature range 0.15K to 2.2K were used to construct a Dm - T phase diagram shown in Figure 4. An open triangle marks the value of Dm at the steepest point on the prewetting step and an open circle marks the position of the dissipation peak on the forward isotherm at each temperature. Corresponding solid symbols mark these features on the reverse isotherms. Some of the scatter in the points in Figure 4 is due to variations among the 3 Rb surfaces; typical behavior of forward isotherms at T=1.22 K for each of the 3 surfaces is shown in Figure 5. Note that the peak occurs at approximately the same value of Dm for each surface, but the size and shape of the peak varies from surface to surface. 
 
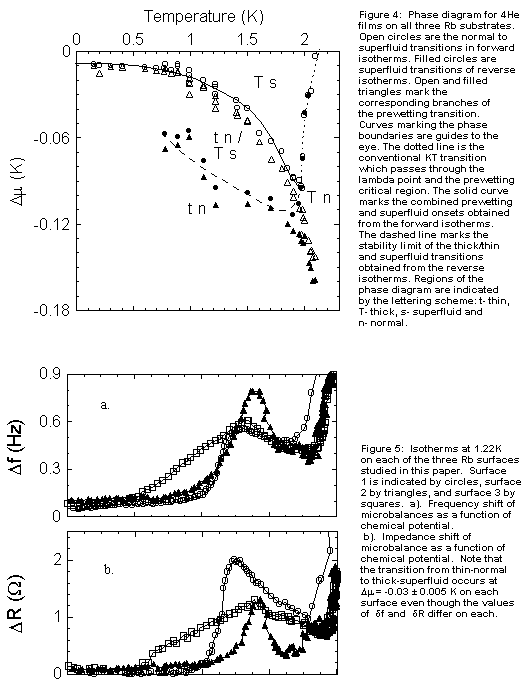
figures 4 & 5 

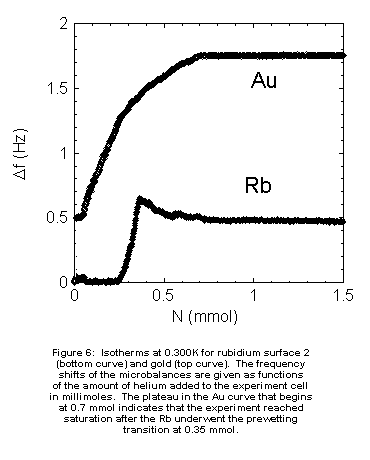
figure 6The solid line in Figure 4 connects the thin->thick transitions of the forward isotherms and will be referred to as the prewetting line. Notice that it does not intersect the liquid-vapor saturation line, Dm = 0, implying that helium wets our rubidium surfaces at all temperatures. This conclusion does not depend on subtleties of our method of measuring Dm at low temperature. After prewetting and superfluid onset, more gas must be added to the cell to reach saturation even at the lowest temperatures. This can clearly be seen in Figure 6, which shows a plot of Df as a function of the amount of gas admitted to the cell, N, for both the rubidium coated oscillator and the bare gold oscillator at T=0.3K. The plateau in Df vs. N for the gold oscillator indicates a region of bulk liquid-vapor coexistence in which the adsorbed film has reached its asymptotic gravitationally limited thickness. The prewetting peak on the Rb oscillator occurs at substantially lower N than the coexistence value. 
It is interesting to inquire whether the Kosterlitz-Thouless picture, which successfully describes superfluid onset on both conventional substrates and Cs, can also account for the combined superfluid/prewetting transition we observe on Rb. We find that although the basic features of a superfluid jump and enhanced dissipation clearly exist, the universal KT predictions do not apply to superfluid onset on Rb. Although the Kosterlitz-Thouless theory makes no explicit prediction about the absolute thickness of the film at superfluid onset, the jump in the superfluid fraction ss depends only on T and universal constants. [2] : 
 
sS(TC) = 8 p m kB TC / h2 (2)

where m is the mass of a helium atom, kB is Boltzmann’s constant, TC is the transition temperature and sS is the coverage in atoms/m2. In terms of our experimental observables, this implies that the frequency shift at onset df is numerically given by 
 

df = -4 fn2 m sS(TC) / n Rq 
 =0.1584 Hz / K × TC		 (3)

where fn is the resonant frequency of the nth harmonic and Rq is the transverse acoustic impedance of quartz (Rq~ 8.862 × 106 kg/m2sec). This prediction is manifestly independent of the substrate. Furthermore, since the KT transition is a higher order transition, it cannot be superheated or supercooled, so no hysteresis in the onset temperature is expected. 
figure 7In our isotherms, the abrupt step in Df on the thick film side of the prewetting transition, denoted by df in Figure 3, can be attributed to a KT-like jump in the superfluid density.  df does not, however, obey the KT prediction of Eq. 2.   For example, the data at 1.50K (Figure 3) shows a superfluid jump of 0.35 Hz (1.4 layers) on the forward isotherm, while the reverse isotherm shows a jump of 0.18 Hz (0.7 layers). The fact that these two values are different is a violation of the KT prediction that the size of the jump is a unique function of T. Furthermore, neither of them is equal to the predicted value of 0.25 Hz (1.0 layer). A number of isotherms like Figure 3 have been used to construct a plot of the magnitude of the superfluid jump at onset, df, as a function of T for both forward and reverse branches on Rb for surfaces 2 & 3, as shown in Figure 7. The universal KT prediction (straight line from Eq. 2) and the df vs. T results from the gold oscillator, determined by the same method we used on the Rb oscillator, are shown for comparison. The agreement between the gold data and the KT prediction is typical of all previously studied surfaces [9,16,18, 25], and provides a check of our experimental procedure. 
The temperature dependence of the dissipation peak associated with superfluid onset on Rb is also anomalous. The forward and reverse dR measurements on Rb for surfaces 1 and 2 and the dR values on Au are shown in Figure 8. The dynamic KT prediction for the dissipation, although not universal, is proportional to T [3]; the expected straight line behavior is also shown in Figure 8. The reverse Rb dissipation peaks and the peaks on Au are similar and conform with the KT picture. However, the forward isotherm dR values grow rapidly with decreasing temperature and in fact appear to be diverging as T goes toward 0 K. Although the absolute value of the loss is different for the two surfaces, the trend of increasing loss with decreasing temperature is seen on both. 
On a conventional substrate, the minimum helium coverage required for superfluidity is considerably higher than the KT critical coverage defined in Eq. 2. This phenomenon is attributed to the existence of a solid-like “dead layer” which is immobilized by the substrate potential. This layer is typically about two monolayers thick, but on weak substrates such as solid hydrogen, the critical coverage for superfluidity at T=0 is approximately 1/2 layer [26-28]. Rb, which is even weaker than H2, is expected to have a very low critical coverage at low temperature. In contrast to this expectation, our data seem to indicate that more than two layers of 4He can remain in the normal state on Rb even at T=0. 
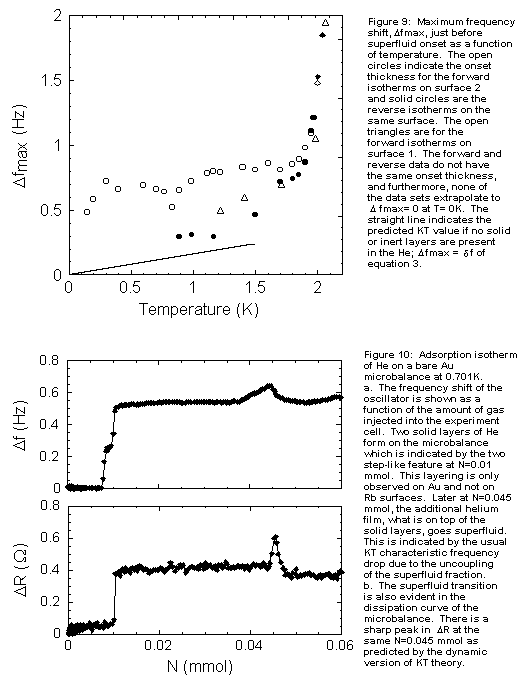
The maximum frequency shift Dfmax associated with the prewetting steps shown in Figure 3 is a convenient measure of the normal coverage at superfluid onset. Figure 9 shows a plot of Dfmax for both forward and reverse isotherms as a function of temperature for surfaces 1 and 3. The solid straight line shows the expected value of df from the KT theory Eq. 3 which is equal to Dfmax if there is no dead layer. Like all of the other features of the isotherms, Dfmax is hysteretic. On the reverse isotherms, Dfmax seems to approach the KT line at the lowest accessible temperatures. 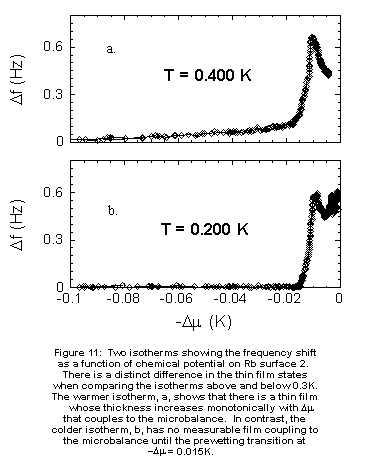On the forward isotherms, Dfmax has almost the same temperature dependence as the KT prediction, but it extrapolates to a remarkably high value of 2.0 layers at T=0. This can be compared to the typical onset behavior on gold, illustrated in Figure 10, which shows two steps corresponding to the formation of 2 (presumably solid) layers followed by superfluid onset at essentially the same value of Dfmax we observe on Rb. 
figures 9 & 10 

figure 11Discussion thus far has focussed on the thin to thick transitions, but there also appears to be a qualitative change in behavior of the thin film phase which occurs near 0.3K and may conceivably be related to the transition reported by Wyatt et al. [13].   Figure 11 shows the frequency shift for two isotherms at 0.2K and 0.4K for surface 2. Both isotherms show a prewetting step and superfluid onset, but the thin phase (Dm<-0.017K) is quite different in the two cases.   The 0.2K isotherm shows no shift in the frequency from the vacuum value until the prewetting step, while the 0.4K isotherm has a continuously increasing value of Df and a frequency shift of 0.2 Hz (corresponding to 0.8 layer) at the beginning of the prewetting transition. One possible explanation of this behavior is a superfluid [13] or other type of phase transition in the thin phase. Another possibility is that the observed differences are due to the fact that the saturated vapor pressure varies by more than a factor of 107 between 0.2K and 0.4K, resulting in significantly different equilibrium times. To test the possible role of mass transfer kinetics, we measured isochores with both increasing and decreasing temperatures.   We began with a vacuum at 0.55K and added just enough helium to the cell to increase the chemical potential to a value below the prewetting transition so the adsorbed film would still be in a thin phase. 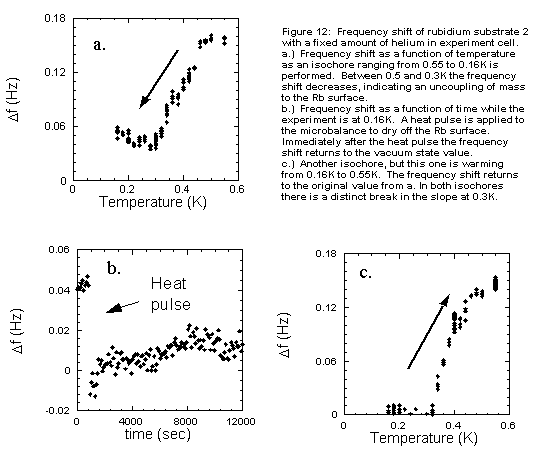The experiment was then cooled down to 0.2K with this fixed amount of helium in the experiment (Figure 12a). As the experiment cooled, the frequency shift decreased indicating that the coupled mass to the microbalance was changing. This could either be due to helium leaving the microbalance or a transition to a thin superfluid film; it cannot be attributed to a mass transfer bottleneck, since the normal film was present at high temperature. The film could be removed from the oscillator by applying a heat pulse to the microbalance at 0.2K, as shown in Figure 12b. The frequency shift remained close to zero for several hours after the heat pulse. The temperature of the experiment was subsequently increased to 0.55K and the microbalance frequency returned to its initial value as shown in Figure 12c. In both the heating and cooling cycles, 0.3K marks a change in slope of Df. 

figure 12